heureka

(-81)^\frac{1}{4}

$\begin{array}{|rcll|} \hline x&=& \sqrt[4]{-81} \\ &=& (-81)^\frac{1}{4} \\ &=& (81)^\frac{1}{4} \cdot (-1)^\frac{1}{4} \\ &=& 3 \cdot (-1)^\frac{1}{4} \quad & | \quad e^{i\pi} = -1\ ! \quad (\text{Euler's Formula for Complex Numbers}) \\ x_1 &=& 3\cdot (e^{i\pi})^\frac{1}{4} \\ &=& 3\cdot e^{i\frac{\pi}{4}} \\ &=& 3\cdot [\cos(\frac{\pi}{4}) + i\cdot \sin(\frac{\pi}{4})] \quad & | \quad \cos(\frac{\pi}{4})=\sin(\frac{\pi}{4})= \frac{\sqrt{2}}{2} \\ &=& 3\cdot (\frac{\sqrt{2}}{2}+ i\cdot \frac{\sqrt{2}}{2} ) \\ &=& \frac{3 \sqrt{2}}{2}(1+i) \\\\ x_2 &=& 3\cdot e^{i(\frac{\pi}{4}+\frac{\pi}{2} )} \\ x_2 &=& 3\cdot e^{\frac{3}{4} i \pi } \\\\ x_3 &=& 3\cdot e^{i(\frac{\pi}{4}+2\cdot\frac{\pi}{2} )} \\ x_3 &=& 3\cdot e^{\frac{5}{4} i \pi } \\\\ x_4 &=& 3\cdot e^{i(\frac{\pi}{4}+3\cdot\frac{\pi}{2} )} \\ x_4 &=& 3\cdot e^{\frac{7}{4} i \pi } \\\\ \hline \end{array}$

Hello CPhill,

i think this is a good method to check the calculation.

Geometry Help

Given a simple polygon constructed on a grid of equal-distanced points (i.e., points with integer coordinates) such that all the polygon's vertices are grid points, Pick's theorem provides a simple formula for calculating the area A of this polygon in terms of the number i of lattice points in the interior located in the polygon and the number b of lattice points on the boundary placed on the polygon's perimeter:

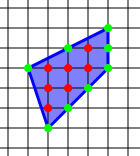
i = 7, b = 8,
A = i + b/2 − 1 = 10

see matrix-subtraction-calculator:

A track team has 121people. The ratio of boys to girls is 7:4.

How many more boys are there on the team than girls?

$\begin{array}{|rcll|} \hline \frac{7}{11} + \frac{4}{11} &=& 1 \quad & | \quad \cdot 121 \\ \frac{7}{11}\cdot 121 + \frac{4}{11}\cdot 121 &=& 121 \\ \frac{7}{11}\cdot 11\cdot 11 + \frac{4}{11}\cdot 11\cdot 11 &=& 121 \\ 7\cdot 11 + 4\cdot 11 &=& 121 \\ \underbrace{77}_{\text{boys}} + \underbrace{44}_{\text{girls}} &=& 121 \\\\ && \text{boys} - \text{girls} \\ &=& 77-44 \\ &=& 33 \\ \hline \end{array}$

Complex root:

$\begin{array}{|rcll|} \hline x &=&\sqrt{1+i}\\ \ln x &=& \dfrac{1}{2}\cdot \ln(1+i)\\ 2\cdot \ln x &=& \ln(1+i)\\ &=& \ln(|1+i|)+i\arg(1+i) \quad & | \quad |1+i| = \sqrt{1^2+1^2}= \sqrt{2} \\ &=& \ln(\sqrt{2})+i\cdot \arg(1+i) \quad & | \quad \arg(1+i) = \arctan(\frac{1}{1}) = \dfrac{\pi}{4} \\ &=& \ln(\sqrt{2}) + i\cdot \dfrac{\pi}{4} \\ 2\cdot \ln x &=&\ln(\sqrt{2}) + i\cdot \dfrac{\pi}{4} \quad & | \quad :2 \\ \ln x &=& \dfrac12 \cdot \ln(\sqrt2) + i\frac{\pi}{8} \\ &=& \ln\left(\sqrt{\sqrt{2}}\right) + i \frac{\pi}{8} \\ \ln x &=& \ln(\sqrt[4]{2}) + i \frac{\pi}{8} \\ x &=&e^{\ln(\sqrt[4]{2}) + i \frac{\pi}{8}} \\ &=&e^{\ln(\sqrt[4]{2})} e^{i \frac{\pi}{8}} \\ x &=& \sqrt[4]{2} \cdot e^{i \frac{\pi}{8}} \\ x_1 &=& \sqrt[4]{2} \cdot (\cos{\frac{\pi}{8}} +i \cdot \sin{\frac{\pi}{8}} )\\ && \cos{\frac{\pi}{8}} = \frac12\cdot \sqrt{2+\sqrt{2}} \\ && \sin{\frac{\pi}{8}} = \frac12\cdot \sqrt{2-\sqrt{2}} \\ x_1 &=& \sqrt[4]{2} \cdot \left( \frac12\cdot \sqrt{2+\sqrt{2}} +i \cdot \frac12\cdot \sqrt{2-\sqrt{2}} \right) \\ \mathbf{x_1} &\mathbf{=}& \mathbf{\sqrt[4]{2}\cdot \frac{\sqrt{2+\sqrt{2}}}{2} +i \cdot \sqrt[4]{2}\cdot \frac{\sqrt{2-\sqrt{2}}}{2} } \\\\ x_2 &=& - x_1 \\ \mathbf{x_2} &\mathbf{=}& \mathbf{-\sqrt[4]{2}\cdot \frac{\sqrt{2+\sqrt{2}}}{2} - i \cdot \sqrt[4]{2}\cdot \frac{\sqrt{2-\sqrt{2}}}{2} } \\ \hline \end{array}$

$x_1 \approx 1.09868 + 0.45509i \\ x_2 \approx -1.09868 - 0.45509i$

I am a number less than 3000.

If you divide me by 32 you will get a remainder 30.

If you divide me by 54 , you will get a remainder 44.

What number am I?

I am 638 or 1502 or 2366

$\begin{array}{rcll} n \equiv {\color{red}30} \pmod {{\color{green}32}} \\ n \equiv {\color{red}44} \pmod {{\color{green}54}} \\\\ \text{or }\quad \begin{array}{rcll} n-30 = 32 r \\ n-44 = 54 s \\ \end{array} \end{array}$

Because 32r or 54s is even
so n-30 or n-44 is even so n is even

We can set: $m = \frac{n}{2}$ and m is an integer.

$\begin{array}{|rcll|} \hline n-30 = 32 r \quad & | \quad :2 \\ \frac{n}{2}-15 = 16 r \\ m-15 = 16 r \\\\ n-44 = 54 s \quad & | \quad :2 \\ \frac{n}{2}-22 = 27 s \\ m-22 = 27 s \\\\ && \text{or }\quad \begin{array}{rcll} m \equiv {\color{red}15} \pmod {{\color{green}16}} \\ m \equiv {\color{red}22} \pmod {{\color{green}27}} \\ \end{array} \\ && \quad \text{ with } n=2m \text{ and } 16\cdot 27 = 432 \\ \hline \end{array}$

Because 16 and 27 are relatively prim ( gcd(16,27) = 1! ) we can go on:

$\begin{array}{rcll} m &=& {\color{red}15} \cdot {\color{green}27} \cdot [ \frac{1}{\color{green}27}\pmod {{\color{green}16}} ] + {\color{red}22} \cdot {\color{green}16} \cdot [ \frac{1}{\color{green}16}\pmod {{\color{green}27}} ] \\ \end{array}$

$\begin{array}{rcll} && [ \frac{1}{\color{green}27}\pmod {{\color{green}16}} ] \\ &=& {\color{green}27}^{\varphi({\color{green}16})-1} \pmod {{\color{green}16}} \\ &=& 27^{8-1} \mod {16} \\ &=& 27^{7} \mod {16} \\ &=& 3 \\\\ && [ \frac{1}{\color{green}16}\pmod {{\color{green}27}} ] \\ &=& {\color{green}16}^{\varphi({\color{green}27})-1} \pmod {{\color{green}27}} \\ &=& 16^{18-1} \mod {27} \\ &=& 16^{17} \mod {27} \\ &=& 22 \\ \end{array}$

$\begin{array}{rcll} m &=& {\color{red}15} \cdot {\color{green}27} \cdot 3 + {\color{red}22} \cdot {\color{green}16} \cdot 22 \\ m &=& 8959 + k\cdot 432 \quad & | \quad k \in Z \\\\ n &=& 2m \\ n &=& 2\cdot(8959 + k\cdot 432 ) \\ n &=& 17918 + k\cdot 864 \\\\ n_{min} &=& 17918 \pmod {864 } \\ n_{min} &=& 638 \\ \mathbf{n} &\mathbf{=}& \mathbf{638 + k\cdot 864} \quad & | \quad k \in Z \\ \end{array}$

$\begin{array}{|lrcll|} \hline k=0: & \mathbf{n} &\mathbf{=}& \mathbf{638} \\ k=1: & n &=& 638+1\cdot 864 \\ & \mathbf{n} &\mathbf{=}& \mathbf{1502} \\ k=2: & n &=& 638+2\cdot 864 \\ & \mathbf{n} &\mathbf{=}& \mathbf{2366} \\ \hline \end{array}$

Two pipes together drain a wastewater holding tank in 6 hours.
If used alone to empty the tank, one takes 2 hours longer than the other.
How long does each take to empty the tank when used alone?

$\begin{array}{|rcll|} \hline \text{Let } q_1 &=& \text{Volumetric flow pipe } 1 \\ \text{Let } q_2 &=& \text{Volumetric flow pipe } 2 \\ \text{Let } V &=& \text{Volume of the tank} \\ \hline \end{array}$

$\begin{array}{|lrcll|} \hline & q_1 \cdot t + q_2 \cdot t &=& V \\ & (q_1 + q_2) \cdot t &=& V \quad & | \quad t = 6\ hours \\ (1) & (q_1 + q_2) \cdot 6 &=& V \\ \hline \end{array}$

$\begin{array}{|lrcll|} \hline (2) & q_1\cdot t_1 &=& V \\ & t_1 &=& \frac{V}{q_1} \\ \hline & q_2 \cdot t_2 &=& V \quad & | \quad t_2 = t_1 + 2\ hours \\ (3) & q_2 \cdot (t_1 + 2) &=& V \\ & q_2 \cdot (t_1 + 2) &=& V \quad & | \quad t_1 = \frac{V}{q_1} \\ & q_2 \cdot (\frac{V}{q_1} + 2) &=& V \\ & \dots \\ (4) & V &=& \frac{2q_1q_2}{q_1-q_2} \\ \hline \end{array}$

(1) = (4):

$\begin{array}{|lrcll|} \hline & V = 6\cdot (q_1+q_2) &=& \frac{2q_1q_2}{q_1-q_2} \\ & 6\cdot (q_1+q_2) &=& \frac{2q_1q_2}{q_1-q_2} \quad & | \quad : 2\\ & 3\cdot (q_1+q_2) &=& \frac{q_1q_2}{q_1-q_2} \quad & | \quad \cdot (q_1-q_2) \\ & 3\cdot (q_1+q_2)(q_1-q_2) &=& q_1q_2 \\ & 3\cdot (q_1^2-q_2^2) &=& q_1q_2 \\ & 3q_1^2-3q_2^2-q_1q_2 &=& 0 \\ & 3q_1^2-q_1q_2-3q_2^2 &=& 0 \\\\ & q_1 &=& \frac{q_2\pm \sqrt{q_2^2-4\cdot 3 \cdot (-3q_2^2) } } {2\cdot 3} \\ & q_1 &=& \frac{q_2\pm \sqrt{ q_2^2+36q_2^2 } } {6} \\ & q_1 &=& \frac{q_2\pm \sqrt{ 37q_2^2 } } {6} \\ & q_1 &=& \frac{q_2\pm q_2\sqrt{37} } {6} \\ & q_1 &=& \frac16(1\pm\sqrt{37} ) q_2\\\\ & \mathbf{q_1 = \frac16(1+\sqrt{37} ) q_2 } &\text{ or }& q_1 = \frac16(1-\sqrt{37} )q_2 \\ & & & \text{ no solution } q_1 < 0 ! \\ (5) & \mathbf{\frac{q_1}{q_2} = \frac16(1+\sqrt{37} ) } \\ \hline \end{array}$

(2) = (3):

$\begin{array}{|lrcll|} \hline & V = q_1\cdot t_1 &=& q_2 \cdot (t_1 + 2) \\ & q_1\cdot t_1 &=& q_2 \cdot (t_1 + 2) \\ (6) & \frac{q_1}{q_2 } &=& \frac{t_1 + 2}{t_1} \\ \hline \end{array}$

(5) = (6):

$\begin{array}{|rcll|} \hline \frac{q_1}{q_2} = \frac16( 1+\sqrt{37} ) &=& \frac{t_1 + 2}{t_1} \\ \frac16( 1+\sqrt{37} ) &=& \frac{t_1 + 2}{t_1} \\ (1+\sqrt{37} )\cdot t_1 &=& 6\cdot(t_1 + 2) \\ (1+\sqrt{37} )\cdot t_1 &=& 6\cdot t_1 + 12 \\ (1+\sqrt{37} -6)\cdot t_1 &=& 12 \\ (\sqrt{37} - 5)\cdot t_1 &=& 12 \\ t_1 &=& \frac{12}{\sqrt{37} - 5} \\ t_1 &=& \frac{12}{6.08276253030- 5} \\ t_1 &=& \frac{12}{1.08276253030} \\ \mathbf{ t_1 } &\mathbf{=}& \mathbf{11.0827625303\ hours }\\\\ t_2 &=& t_1 + 2\ hours \\ \mathbf{ t_2 } &\mathbf{=}& \mathbf{13.0827625303\ hours }\\ \hline \end{array}$

Use prime factorization to find the cube root of 3375

$\begin{array}{|rcll|} \hline && \sqrt[3]{3375} \\ &=& \sqrt[3]{3^3\cdot 5^3} \\ &=& \sqrt[3]{3^3} \cdot \sqrt[3]{ 5^3} \\ &=& 3 \cdot 5 \\ &=& 15 \\ \hline \end{array}$

Find the sum of 35 terms of an arithmetic series
of which the first term is a and the fifteenth term is 9a.

Let t₁ = a

Let t₁₅ = 9a

Formula:

$\begin{array}{|lrcll|} \hline (1) & t_x &=& t_1 + (x-1)\cdot d \\ (2) & t_y &=& t_1 + (y-1)\cdot d \\ (3) & t_z &=& t_1 + (z-1)\cdot d \\ \hline I = (1) - (2) & t_x-t_y &=& d\cdot (x-y) \\ II = (1) - (3) & t_x-t_z &=& d\cdot (x-z) \\ \hline II / I & \frac{t_x-t_z}{t_x-t_y} &=& \frac{d\cdot (x-z)}{d\cdot (x-y)} \\ & \frac{t_x-t_z}{t_x-t_y} &=& \frac{x-z}{x-y} \quad & | \quad \cdot (t_x-t_y) \\ & t_x-t_z &=& (t_x-t_y)\cdot \frac{x-z}{x-y} \\ & t_z &=& t_x-(t_x-t_y)\cdot \frac{x-z}{x-y} \\ & t_z &=& t_x- t_x\cdot (\frac{x-z}{x-y}) +t_y\cdot (\frac{x-z}{x-y}) \\ & t_z &=& t_x\cdot (1-\frac{x-z}{x-y}) +t_y\cdot (\frac{x-z}{x-y}) \\ & t_z &=& t_x\cdot (\frac{x-y-x+z}{x-y}) +t_y\cdot (\frac{x-z}{x-y}) \\ &\mathbf{ t_z } &\mathbf{=}& \mathbf{ t_x\cdot (\frac{z-y}{x-y}) +t_y\cdot (\frac{x-z}{x-y}) } \\ \hline \end{array}$

t₃₅ = ?

$\begin{array}{|lrclrcl|} \hline x = 1 \quad & t_x &=& t_1 = a \\ y = 15 \quad & t_y &=& t_{15} = 9a \\ z = 35 \quad & t_z &=& t_{35} = \ ? \\ &&&& \mathbf{ t_z } &\mathbf{=}& \mathbf{ t_x\cdot (\frac{z-y}{x-y}) +t_y\cdot (\frac{x-z}{x-y}) } \\ &&&& t_{35} & = & a\cdot ( \frac{35-15}{1-15}) + 9a\cdot (\frac{1-35}{1-15}) \\ &&&& t_{35} & = & a\cdot (\frac{20}{-14}) + 9a\cdot (\frac{-34}{-14}) \\ &&&& t_{35} & = & -a\cdot (\frac{10}{7}) + 9a\cdot (\frac{17}{7}) \\ &&&& t_{35} & = & 9a\cdot (\frac{17}{7}) -a\cdot (\frac{10}{7}) \\ &&&& t_{35} & = & a\cdot (\frac{9\cdot 17}{7}) -a\cdot (\frac{10}{7}) \\ &&&& t_{35} & = & a\cdot (\frac{153}{7}) -a\cdot (\frac{10}{7}) \\ &&&& t_{35} & = & a\cdot (\frac{153-10}{7}) \\ &&&& \mathbf{ t_{35} } &\mathbf{=}& \mathbf{ \frac{143}{7}a }\\ \hline \end{array}$

s₃₅ = ?

$\begin{array}{|rcll|} \hline s_{35} &=& \left(\frac{t_1+t_{35}}{2} \right)\cdot 35 \quad & | \quad t_1 = a \quad t_{35} = \frac{143}{7}a \\ s_{35} &=& \left(\frac{a+\frac{143}{7}a}{2} \right)\cdot 35 \\ s_{35} &=& \left(\frac{1+\frac{143}{7} }{2} \right)\cdot 35 a\\ s_{35} &=& \left( \frac{150}{14} \right)\cdot 35 a\\ s_{35} &=& 150\cdot \frac{5}{2} a\\ s_{35} &=& 75\cdot 5 a\\ \mathbf{ s_{35} } &\mathbf{=}& \mathbf{375 a}\\ \hline \end{array}$