hectictar

$\begin{array}{} \phantom{=\qquad}\frac{\cos(90°+x)}{\cos(360°-x)\tan(180°-x)\cos(480°)}&\\~\\ =\qquad\frac{-\sin(x)}{\cos(360°-x)\tan(180°-x)\cos(480°)}&\qquad\text{because}\qquad \cos(90°+x)=-\sin (x) \\~\\ =\qquad\frac{-\sin(x)}{\cos(-x)\tan(180°-x)\cos(480°)}&\qquad\text{because}\qquad \cos(360°-x)=\cos(-x+360°)=\cos(-x) \\~\\ =\qquad\frac{-\sin(x)}{\cos(x)\tan(180°-x)\cos(480°)}&\qquad\text{because cos(x) is an even function,}\quad \cos(-x)=\cos(x) \\~\\ =\qquad\frac{-\sin(x)}{\cos(x)(-\tan(x))\cos(480°)}&\qquad\text{because}\qquad \tan(180°-x)=-\tan(x)\\~\\ =\qquad\frac{\sin(x)}{\cos(x)\tan(x)\cos(480°)}&\\~\\ =\qquad\frac{\sin(x)}{\cos(x)\tan(x)\cos(120°)}&\qquad\text{because}\qquad \cos(480°)=\cos(480°-360°)=\cos(120°) \end{array}$

So far the only difference is because  tan(180° - x)  =  -tan(x)

$\begin{array}{} \phantom{=\qquad}\frac{\sin(x)}{\cos(x)\tan(x)\cos(120°)}&\\~\\ =\qquad\frac{\sin(x)}{\cos(x)}\cdot\frac{1}{\tan(x)\cos(120°)}&\\~\\ =\qquad\tan(x)\cdot\frac{1}{\tan(x)\cos(120°)}&\qquad\text{because}\qquad \frac{\sin(x)}{\cos(x)}=\tan(x)\\~\\ =\qquad\frac{1}{\cos(120°)}&\\~\\ =\qquad\frac{1}{(-\frac12)}&\qquad\text{because}\qquad \cos(120°)=-\frac12\\~\\ =\qquad-2& \end{array}$

The domain and range are both sets. If you are listing all the elements in a set, then the order that you list the elements does not matter.

The set  {2, 3, 1, 5}  is the same as the set  {1, 2, 3, 5} .

So no, you do not need to list the elements in numerical order.

-2x²  =  -(5x + 4)

                               Distribute  -1  to the terms in parenthesees.

-2x²  =  -5x - 4

                               Add  2x²  to both sides of the equation.

0  =  2x² - 5x - 4

2x² - 5x - 4  =  0

2x² + -5x + -4  =  0

This is a quadratic equation. We can use the quadratic formula to solve for  x .

The quadratic formula says that....

If     ax² + bx + c  =  0     then     $x=\frac{-\mathbf{\color{blue}b}\pm\sqrt{\mathbf{\color{blue}b}^2-4\mathbf{\color{Magenta}a}\mathbf{\color{Green}c}}}{2\mathbf{\color{Magenta}a}}$

So...

Since     2x² + -5x + -4  =  0     then     $x=\frac{-(\mathbf{\color{blue}-5})\pm\sqrt{\mathbf{(\color{blue}-5})^2-4(\mathbf{\color{Magenta}2})(\mathbf{\color{Green}-4})}}{2(\mathbf{\color{Magenta}2})}$

$x=\frac{-(-5)\pm\sqrt{(-5)^2-4(2)(-4)}}{2(2)}\\~\\ x=\frac{5\pm\sqrt{25+32}}{4}\\~\\ x=\frac{5\pm\sqrt{57}}{4}\\~\\ x=\frac{5+\sqrt{57}}{4}\qquad\text{or}\qquad x=\frac{5-\sqrt{57}}{4}$

The possible values of  n  are:

1, 5, 7, 11, 13, 17, 19, 23, 25, 29, 31, 35, 37, 41, 43, 47

And the elements in the set are:

$\frac{1}{24}, \frac{5}{24}, \frac{7}{24}, \frac{11}{24}, \frac{13}{24}, \frac{17}{24}, \frac{19}{24}, \frac{23}{24}, \frac{25}{24}, \frac{29}{24}, \frac{31}{24}, \frac{35}{24}, \frac{37}{24}, \frac{41}{24}, \frac{43}{24}, \frac{47}{24}$

So the sum of the elements in the set   =   $\frac{384}{24}$   =   16

Draw WY to create △WXY .

△WXY is an isoscelese right triangle so the measure of each base angle is  45°

The length of each leg of  △WXY  is  4  so the length of the hypotenuse is  4√2

m∠XYZ  =  135°

m∠WYZ  =  m∠XYZ - m∠WYX  =  135° - 45º  =  90°

So  △WYZ  is a right triangle, and we know the length of two of the sides.

To find the length of the missing side, we can use the Pythagorean theorem.

a²  +  ( 4√2 )²   =   9²

a²  +  32   =   81

                                Subtract  32  from both sides.

a²   =  49

                                Take the positive sqrt of both sides.

a  =  7

y  =  x² + bx + c

The graph of the equation passes through the point  (-1, -11)  so we know....

-11  =  (-1)² + b(-1) + c

-11  =  1 - b + c

                                 Add  b  to both sides.

-11 + b  =  1 + c

                                 Add  11  to both sides.

b  =  12 + c

The graph of the equation passes through the point  (3, 17)  so we know....

17  =  (3)² + b(3) + c

17  =  9 + 3b + c

                                 Subtract  9  from both sides.

8  =  3b + c

                                 And we know  b  =  12 + c  so we can substitute  12 + c  in for  b .

8  =  3(12 + c) + c

                                 Distribute  3  to the terms in parenthesees.

8  =  36 + 3c + c

                                 Subtract  36  from both sides.

8 - 36  =  3c + c

                                 Combine like terms.

-28  =  4c

                                 Divide both sides by  4 .

-7  =  c

Part of this last problem is just like the second problem.......

In the diagram below, we know  $\tan\theta=\frac34$ . Find the area of the triangle.

Let the base of the triangle be the side with length 60. Draw a height to that base and call it  h .

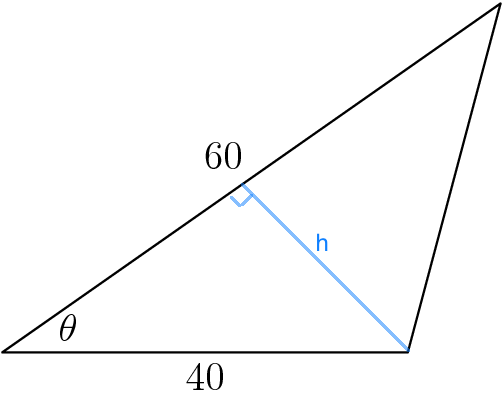
By the Pythagorean Identity,

Find the value of   $\sin40°\cdot\cos50°+\cos40°\cdot\sin50°$ .

Remember the angle addition formula for sine:    $\sin(\alpha+\beta)\ =\ \sin\alpha\cos\beta+\cos\alpha\sin\beta$

So...

$\sin40°\cdot\cos50°+\cos40°\cdot\sin50°\ =\ \sin(40°+50°)\ =\ \sin(90°)\ =\ 1$

_ _ _ _ _

In $\triangle PQR,$ we have $\angle P = 90^\circ,$ $PQ=3,$ and $QR=\sqrt {58}.$ Find $\tan R.$

By the Pythagorean theorem,

3² + ( PR )²  =  √[ 58 ]²

9  +  ( PR )²  =  58

( PR )²  =  49

PR  =  7

tan( angle )  =  opposite / adjacent

tan( R )  =  3 / PR

tan( R )  =  3 / 7

Find AC in the diagram to the nearest integer.

sin( angle )  =  opposite / hypotenuse

sin( 70° )  =  31 / AC

                                         Multiply both sides of the equation by  AC

AC sin( 70° )  =  31

                                         Divide both sides of the equation by  sin( 70° )

AC  =  31 / sin( 70° )

                                         Plug    31 / sin( 70° )    into a calculator.

AC  ≈  33